ข้อสอบ ACT Math ล่าสุด พร้อมเฉลยลักษณะของข้อสอบจริง

1. Find the inverse equation of 5x−4y=18.

\displaystyle y=\frac{4x+5}{18}

x = y

\displaystyle y=\frac{4x+18}{5}

\displaystyle y=\frac{x+18}{4}

\displaystyle x=\frac{4y+18}{5}

2. Let F(x) = x3 + 2x2 – 3 and G(x) = x + 5. Find F(G(x))

x^3 + x^2 + 2

x^3 + 2x^2 - x - 8

x^3 + 2x^2 + x + 2

x^3 + x^2 + x + 8

x^3 + 17x^2 + 95x + 172

3. Where does the following equation intercept the x-axis? y=x2+5x−9

(−1.4,0) and (6.4,0)

(−1.4,0) and (−6.4,0)

(−1.4,0) and (6.4,0)

(0,1.4) and (0,−6.4)

(1.4,0) and (-6.4,0)

4. In the standard coordinate plane, what is the midpoint of a line segment that goes from the point (3, 5) to the point (7, 9)?

(6,6)

(–2, –2)

(10,14)

(7, 5)

(5, 7)

5. We have a square with length 2 sitting in the first quadrant with one corner touching the origin. If the square is inscribed inside a circle, find the equation of the circle.

(x+1)^+(y+1)^2 = 1

x^2 + y^2 = 1

(x−1)^2 + (y−1)^2 = 2

(x−1)^2 + (y−1)^2 = 1

(x-1)^2 + (y-1)^2 = 2

6. Give the equation, in slope-intercept form, of the line tangent to the circle of the equation

x^2 + y^2 = 225
at the point (12,−9).

\displaystyle y = \frac{3} {4}x-18

\displaystyle y = \frac{3} {4}x

\displaystyle y = \frac{4} {3}x+7

None of the other responses gives the correct answer.

\displaystyle y = \frac{4} {3}x-25

7. Which of the following could be a value of x, given the following inequality?
\displaystyle 8-7x\leq11x+4

\displaystyle -\frac{2}{3}

\displaystyle -\frac{2}{9}

\displaystyle -\frac{1}{9}

\displaystyle -\frac{4}{9}

\displaystyle -\frac{1}{3}

8. The length of a rectangular piece of land is two feet more than three times its width. If the area of the land is \displaystyle 125\:ft^2 , what is the width of that piece of land?

\displaystyle \frac{-1+2\sqrt{94}}{3}\:ft^2

\displaystyle \frac{-1-2\sqrt{94}}{3}\:ft^2

\displaystyle \frac{1-2\sqrt{94}}{3}\:ft^2

\displaystyle \frac{-1+4\sqrt{94}}{3}\:ft^2

\displaystyle \frac{2-4\sqrt{94}}{6}\:ft^2

9. The cost of a movie ticket and a candy bar is $5. The cost of two tickets and a candy bar is $8.75. How much is a candy bar?

$2.50

$0.75

$1.25

$7.50

$3.75

10. Simplify the following:
\displaystyle \frac{K^3K^4}{M^6M^2}

\displaystyle \frac{KM^{\frac{1}{2}}}{KM^{\frac{1}{2}}}

\displaystyle \frac{K^8}{M^7}

\displaystyle \frac{K^-1}{M^4}

\displaystyle \frac{K^7}{M^8}

\displaystyle \frac{K^12}{M^12}

11. If m and n< are both rational numbers and \displaystyle 4^{m} = 8^{n} , what is \displaystyle \frac{m}{n}?

\displaystyle \frac{2}{1}

\displaystyle \frac{3}{2}

\displaystyle \frac{5}{3}

\displaystyle \frac{4}{1}

12. Use the FOIL method to simplify the following expression:
\displaystyle (x^3+2x^2)^2

\displaystyle 4x^5+4

\displaystyle x^6+4x^4

\displaystyle 5x^6+4x^4

\displaystyle x^6+4x^5 + 4x^4

\displaystyle x^6+2x^4

13. If \displaystyle \small \log_{x}49=2 , what is x?

7

0

24.5

10

2401

14. What is 0.000000537 in scientific notation?

\displaystyle 5.37\times 10^{-6}

\displaystyle 5.37\times 10^{6}

\displaystyle 5.37\times 10^{-7}

\displaystyle 5.37\times 10^{7}

5.37

15.Simplify: \displaystyle \frac{9+4i}{4i}

\displaystyle \frac{ 9 }{4 } - i

\displaystyle - \frac{ 9 }{4 } + i

\displaystyle \frac{ 9 }{4 } + i

\displaystyle 1- \frac{ 9 }{4 }i

\displaystyle 1+ \frac{ 9 }{4 }i

16. The length in cm of a plastic container is 5cm less than triple its width. Which of the following equations is an accurate description of the length, l, as a function of the width, w?

l = 3w – 5

l = ⁵/₃w + ³/₅

l = ¹/₃w + 5

l = 3w + 5

l = ¹/₃w - 5

17. Simplify the following rational expression:
\displaystyle \frac{5x-5}{2x^{2}} + \frac{7x+9}{2x^{2}}

\displaystyle \frac{8x+4}{2x^{2}}

\displaystyle \frac{6x+4}{2x^{2}}

\displaystyle \frac{6x+8}{2x^{2}}

\displaystyle \frac{12x+8}{2x^{2}}

\displaystyle \frac{6x+2}{x^{2}}

18. Simplify:\displaystyle \begin{bmatrix} 14&22\\ -3 &4 \end{bmatrix}+\begin{bmatrix} -3&12\\ 5 &-10 \end{bmatrix}

\displaystyle \begin{bmatrix} 17 & -5 \\ 3 & 1 \end{bmatrix}

\displaystyle \begin{bmatrix} 13\\ 28 \end{bmatrix}

\displaystyle \begin{bmatrix} 11&34\\ 2 &-6 \end{bmatrix}

\displaystyle \begin{bmatrix} 45&-4 \end{bmatrix}

\displaystyle \begin{bmatrix} 17&10\\ -8 &14 \end{bmatrix}

19. What is a+b?
\displaystyle 5\begin{bmatrix} a \\ b \end{bmatrix} = 14\begin{bmatrix} 20 \\ 12 \end{bmatrix}

\displaystyle \frac{191}{4}

\displaystyle \frac{148}{5}

\displaystyle \frac{448}{5}

29

\displaystyle \frac{41}{5}

20. The price of silver varies directly as the square of the mass. If 3.6 g of silver is worth $64.80, what is the value of 7.5 g of silver?

$281.25

$215.25

$178.50

$135.00

$301.75

21. Choose the answer that is the simplest form of the following expression of monomial quotients:
\displaystyle \frac{12x^3y^2p}{4ab}\div\frac{3x^3y}{2ab}

3ax

2yp

3yp

12xp

2xy

22. Simplify:
\displaystyle \frac{x^2+9x-10}{x-1}

(x−10)

(x+1)

(x+10)

(x+2)

(x−1)

23. Find the degree of the polynomial:
\displaystyle 5x^3+x^2y^6+7y^4

6

13

12

15

8

24.\displaystyle \sqrt{2016} + \sqrt{896} = ?

54

\displaystyle 20\sqrt{14}

\displaystyle 208\sqrt{14}

75

\displaystyle \sqrt{2919}

25. What is the ratio of \displaystyle \sqrt{105} to \displaystyle \sqrt{\frac{12}{5}}

\displaystyle 4\sqrt{7} : 15

\displaystyle 5\sqrt{7} : 2

\displaystyle \sqrt{105} : 12

\displaystyle \sqrt{15} : 4

\displaystyle 5\sqrt{21} : 12

26. Evaluate: \displaystyle \sqrt{-0.64}

−0.4096

−0.04096

−0.8

Answer does not exist.

−0.008

27. Arrange the following from least to greatest: 0.07,14,1.44,0.007,0.08,0.9,1.33

0.007,0.08,0.07,0.9,1.33,1.44,14

14,1.33,1.44,0.08,0.07,0.9,0.007

14,1.44,1.33,0.9,0.08,0.07,0.007

0.007,0.07,0.08,0.9,1.33,1.44,14

28. What does \displaystyle \small \frac{\frac{2}{3}\times \frac{1}{4}}{\frac{2}{9}+\frac{1}{3}} equal?

\displaystyle \frac{5}{54}

\displaystyle \frac{18}{35}

\displaystyle \frac{11}{17}

\displaystyle \frac{1}{2}

\displaystyle \frac{3}{10}

29. On a 15,000 acre plot of land, there are 7 acres in which deer live. What percentage of the land does this represent? Round to the nearest hundredth.

0.25%

0.01%

0.05%

0.04%

0%

30. Convert 2\frac{2}{5} to an improper fraction.

\displaystyle \frac{11}{5}

\displaystyle \frac{9}{5}

\displaystyle \frac{13}{5}

\displaystyle \frac{14}{5}

\displaystyle \frac{12}{5}

31. One serving of party drink is comprised of 3oz of syrup, 4oz of water, and 3oz of apple juice. If a large bowl of the drink contains 237oz of apple juice, how much of the total drink is in the bowl?

790oz

313oz

2730oz

79oz

553oz

32. A car averages 31 miles per gallon. Currently, gas costs $3.69 per gallon. About how much would it cost in gas for this car to travel 3,149 miles?

$853.38

$374.83

$273.52

$101.58

33. Solve \displaystyle |z + 2| \leq -2

No solutions

All real numbers

z=−4

z≤−4

z≤0

34. ab is even abc is even Therefore, which of the following must be true about bc?

It must be odd.

It must be even.

It could be either even or odd.

35. What is the greatest common factor of 72 and 84?

12

6

18

10

36. A sliced cake can be equally split among 4, 5, or 6 people, with each person receiving the same number of slices. Which of the following answers represents a possible number of slices that the cake could have?

116

30

56

120

24

37. Choose the answer which best solves the following equation:
\displaystyle -\frac{396}{x} = 12

x=−33

x=33

x=−23

x=23

x=28

38. Which of the following inequalities is represented by the number line shown above?

\displaystyle |3x - 12| \leq 6

\displaystyle 5x \leq 25

\displaystyle |5x - 5| \geq 25

\displaystyle 4x \geq -8

\displaystyle |4x - 6| \leq 14

39. A student is taking a test consisting of six questions. It is a multiple choice test and each question has four answers labelled A, B, C, and D. How many ways can the student answer all six questions if he does not choose the same answer for any two consecutive questions?

324

30

100

972

625

40. Solve for \displaystyle x:
x = (3-2)^2 +3 - 3\cdot 2^2

\displaystyle x = -4

\displaystyle x = 4

\displaystyle x = -8

\displaystyle x = 0

\displaystyle x = 8

41. There are n pennies in a jar. Let p represent the decimal ratio of the pennies that are dated before 1983. Which of the following equations repesents the number of pennies that are dated 1983 or later?

n(1-p)/100

np

n(1-p)

n(100-p)

42. Amy recently opened a new credit card. In her first month, expenditures totaled $500 and she was not charged any interest. Amy paid $80 from her first month's bill. The second month, Amy spent another $60 on her credit card. This time, she was charged 5% interest on her total unpaid balance. How much interest was Amy charged?

$24.00

$12.00

$48.00

$32.00

$4.80

43. Given a standard deck of 52 playing cards, where half (clubs and spades) are black and half (hearts and diamonds) are red, what is the probability of picking three black cards in a row if the cards are not replaced?

\displaystyle \frac{3}{52}

\displaystyle \frac{6}{23}

\displaystyle \frac{11}{45}

\displaystyle \frac{5}{19}

\displaystyle \frac{2}{17}

44. Angela scores 17, 19, 13, 24, and 14 points in the first five games of a seven-game basketball season. If the scoring leader in Angela’s league averages 18 points per game, how many points must Angela score in the final two games combined to end the season with the highest scoring average in the league AND have a higher scoring average than any other player?

37

32

40

39

34

45. The list below shows a ninth grader's grades for the academic year. What is the difference between the median and the mode of these grades?

67, 73, 85, 83, 80, 73, 94, 65, 80, 73, 98, 59, 76

4

0

3

1

2

46.Giving the Venn diagram above, what is the sum of the numbers in the set A∪C?

23

27

7

37

46

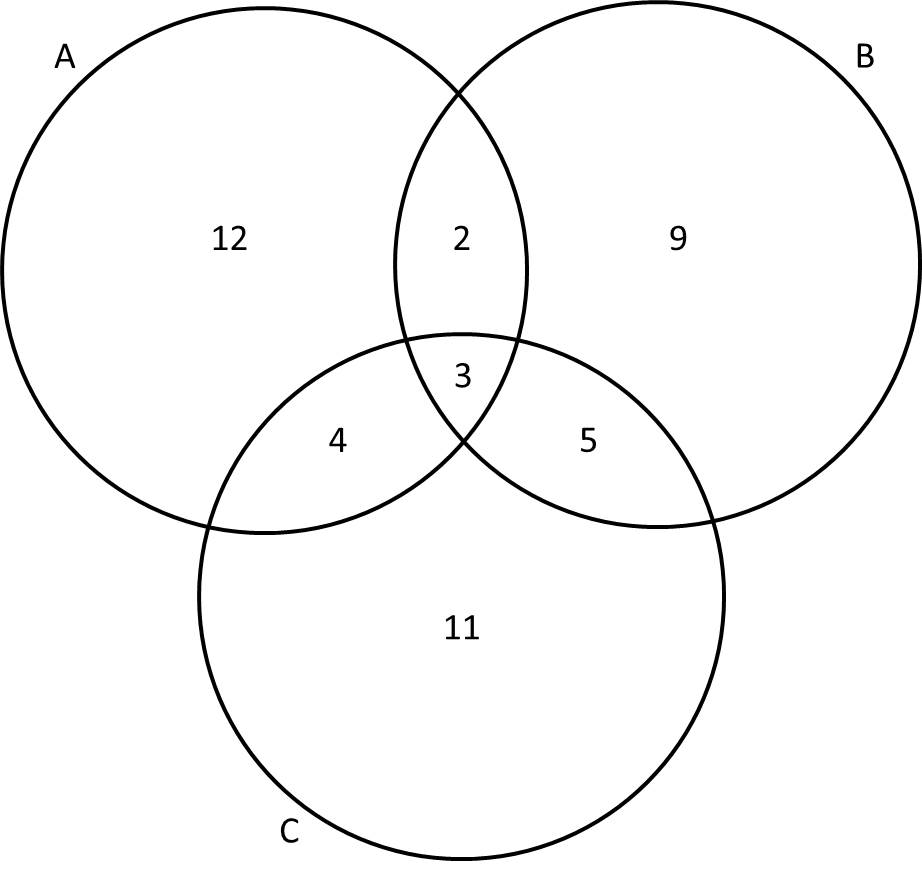
47. Stan is making some giant circular pies for his friend's wedding. The pies each have a diameter of 6ft and will be cut into 64 equal pieces. Stan wants to have three pieces to himself. What is the surface area of the pie that Stan will eat if he eats three pieces?

\displaystyle \frac{7\pi}{15} ft^{2}

\displaystyle \frac{32\pi}{9} ft^{2}

\displaystyle \frac{64\pi}{27} ft^{2}

\displaystyle \frac{27\pi}{64} ft^{2}

\displaystyle \frac{8\pi}{3} ft^{2}

48. What is the value of angle x in the figure above?

\displaystyle 110^{\circ}

\displaystyle 35^{\circ}

\displaystyle 100^{\circ}

\displaystyle 50^{\circ}

\displaystyle 75^{\circ}

49. Find the area of a trapezoid if the height is 2x, and the small and large bases are 2x and 4x, respectively.

\displaystyle 6x

\displaystyle 12x^2

\displaystyle 12x

\displaystyle 6x^2

\displaystyle 16x

50. Two similar triangles' perimeters are in a ratio of 2:5. If the lengths of the larger triangle's sides are 14, 18, and 24, what is the perimeter of the smaller triangle?

21.6

21.4

22.4

22.8

51. What is the perimeter of an equilateral triangle with an area of \displaystyle 56.25\sqrt{3}

\displaystyle 50\sqrt{3}

\displaystyle 45\sqrt{3}

\displaystyle 22\sqrt{3}

15

45

52. An isosceles triangle has a base of 12 cm and an area of 42 cm². What must be the height of this triangle?

10 cm

9 cm

7 cm

8 cm

6 cm

53. A 17 ft ladder is propped against a 15 ft wall. What is the degree measurement between the ladder and the ground?

\displaystyle \cot \left( \frac{15}{8}\right)

\displaystyle \tan^-1 \left( \frac{15}{8}\right)

\displaystyle \cot \left( \frac{8}{15}\right)

\displaystyle \csc \left( \frac{15}{17}\right)

\displaystyle \cos \left( \frac{17}{13}\right)

54. The height of a cone is \displaystyle h; the diameter of its base is twice the height. Give its surface area in terms of \displaystyle h.

\displaystyle 4 \pi h^{2}

\displaystyle \left (1 + \sqrt{2} \right ) \pi h^{2}

\displaystyle \left (2 +2 \sqrt{2} \right ) \pi h^{2}

\displaystyle 5 \pi h^{2}

\displaystyle 10 \pi h^{2}

55. Find the length of the diagonal of a cube with side length of 4cm.

4cm

\displaystyle 4\sqrt{2}cm

6cm

\displaystyle 4\sqrt{3}cm

\displaystyle 2\sqrt{3}cm

56. A spherical plastic ball has a diameter of 4in. What is the volume of the ball to the nearest cubic inch?

\displaystyle 22\:in^3

\displaystyle 56\:in^3

\displaystyle 44\:in^3

\displaystyle 34\:in^3

\displaystyle 269\:in^3

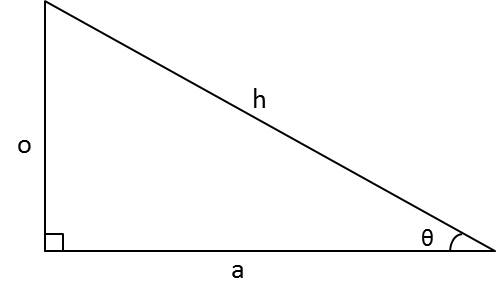
57.For the above triangle, \displaystyle a = 12 and \displaystyle h = 30. Find θ.

\displaystyle 25.6^{\circ}

\displaystyle 21.8^{\circ}

\displaystyle 66.4^{\circ}

\displaystyle 0.4^{\circ}

\displaystyle 68.2^{\circ}

58. What is the sine of the angle formed between the origin and the point (4,−10) if that angle is formed with one side of the angle beginning on the x-axis and then rotating counter-clockwise to (4,−10)?

\displaystyle \frac{5}{\sqrt{29}}

−2.5

2.5

\displaystyle - \frac{5}{\sqrt{29}}

\displaystyle 4\sqrt{10}

59. A man is setting up a laser on the ground, angling it toward the very top of a flag pole. If the flag pole is 20ft high and the laser is placed 12ft away from its base, what should be the angle of the laser with the ground? (Answer in degrees, rounding to the nearest hundredth.)

\displaystyle 3.10^{\circ}

\displaystyle 1.03^{\circ}

\displaystyle 51.49^{\circ}

\displaystyle 59.04^{\circ}

\displaystyle 81.41^{\circ}

60. What is the equation of a line that runs perpendicular to the line 2x + y = 5 and passes through the point (2,7)?

2x + y = 7

–x/2 + y = 6

x/2 – y = 6

2x – y = 6

x/2 + y = 5